KAlgebra/Homework/uk: Difference between revisions
Created page with "===Приклад 1===" |
Created page with "Ми отримаємо результат логічного множення вхідних змінних." |
||
| Line 83: | Line 83: | ||
{{Input|1=and(variable1, variable2)}} | {{Input|1=and(variable1, variable2)}} | ||
Ми отримаємо результат логічного множення вхідних змінних. | |||
===Example 2=== | ===Example 2=== | ||
Revision as of 18:40, 27 April 2011
На цій сторінці наведено приклади застосування KAlgebra для розв’язання конкретних задач.
Приклад з комбінаторики
Нехай слід визначити кількість способів, якими можна розсадити 6 осіб (p1, p2 ... p6) навколо круглого столу з 6 стільцями.
Очевидно, можливі такі варіанти:
p1 p2 p3 p4 p5 p6 p1 p2 p3 p4 p6 p5 p1 p2 p3 p5 p4 p6 p1 p2 p3 p5 p6 p4
і так далі.
Можна зауважити, що останню позицію за столом можна заповнити лише у один спосіб, передостанню — у 2, далі — у 3, у 4, у 5, і нарешті у 6 способів. Отже загальну кількість варіантів можна визначити за допомогою простої формули:
6*5*4*3*2*1
Введемо цю формулу у консоль KAlgebra, і програма обчислить відповідь:
(((((1)*2)*3)*4)*5)*6 =720
Подібний розподіл значень за варіантами, де кількість значень дорівнює кількості варіантів називається «переставляннями».
Ви можете скористатися функцією KAlgebra для обчислення кількості таких переставлянь (ця функція називається «факторіал»):
factorial(6)
дасть нам
factorial(6) =720
Як бачите, ми отримали той самий результат.
Приклад з теорії ймовірностей
Let's roll a dice. We want to know the probability of one face appearing.
We can define positive probability, the result of the event being favourable to us, and negative probability, the result of the event being unfavourable to us.
So you have to pick only one face:
- probability = face picked / total face = 1/6
So now we know that when a dice is rolled there is a 1/6 of probability that a face we chose will come up.
We can set a simple function in KAlgebra to take this formula in a simple way:
probability:=(favorable,total)->favorable/total
Numerical Theory
Let's say that we want to know the sum of all numbers between a bounded interval, for instance 1 - 100. We have to do the sum of all numbers from 0 to 100 if we don't know the rule to get them.
KAlgebra offers a great facility to this task. Let's write in console:
sum(x: x=1..100)
в результаті отримаємо:
sum(x: x=1..100) = 5050
Вказана нами синтаксична конструкція призначена для виконання такої послідовності дій:
- 1. Bound x as variable
- 2. Take first value of x
- 3. Take second value of x and add the previous value of x
- 4. Take third value of x and add the previous value of x
- ...
- N. Take the last value of x and add the last value of x
Розрахунок електричних ланцюгів
Приклад 1
Let's take a simple circuit a and port with two inputs and one output. To resolve it in KAlgebra we will write
and(variable1, variable2)
Ми отримаємо результат логічного множення вхідних змінних.
Example 2
We have a simple circuit: a battery of 3V and two electrical resistances (R1 and R2) put on parallel of 3kOhm. We want to get the current circulating in the circuit.
We have first to calculate the value of the electric resistance expressed according to the law:
- TotalResistance = (1/R1 + 1/R2)^-1
- Current = Voltage/TotalResistance
Let's write a simple function in KAlgebra to do this:
totalresistance:=(R1,R2)->(1/R1+1/R2)^-1
current:=(voltage,totalresistance)->voltage/totalresistance
Let's see what we get:
current(3, totalresistance(3000, 3000))
current(3, totalresistance(3 000, 3 000)) = 0,002
Fluid
Example Problem with Same Material, but Different Volumes and Temperatures
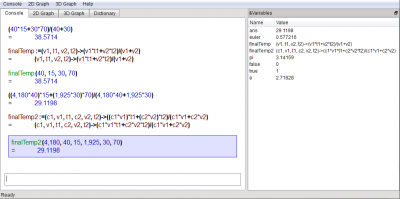
Now, what if we need to know the final temperature when we mix 40L of 15°C water with 30L of 70°C water?
Using conservation of energy, we know that the initial and final thermal energies are the same, so the final energy is equal to the energy of the first fluid plus the energy of the second fluid(using U for internal energy):
- U(final) = U1 + U2
Internal energy is equal to the volumetric heat capacity times volume times temperature:
- U = C*V*T
So C(final)*V(final)*T(final) = C1*V1*T1 + C2*V2*T2
And since the heat capacities are all the same and cancel out, and the final volume is the sum of the two initial volumes:
- (V1+V2)*T(final) = V1*T1 + V2*T2
- or
- T(final) = (V1*T1 + V2*T2)/(V1+V2)
We can then either use this directly in KAlgebra:
(40*15 + 30*70)/(40 + 30)
(40*15+30*70)/(40+30) =38.5714
and get the final temperature, or put in a function if we need to repeat the computation:
finalTemp:=(v1,t1,v2,t2)->(v1*t1 + v2*t2)/(v1+v2)
Which we can then use like this:
finalTemp(40,15,30,70)
finalTemp(40, 15, 30, 70) =38.5714
Example Problem with Different Fluids
Now, suppose the two fluids have different volumetric heat capacities, such as 4180 J/(L*K) for the first liquid (water), and 1925 J/(L*K) for the second liquid (ethanol).
We will need to refer back to the equation:
- C(final)*V(final)*T(final) = C1*V1*T1 + C2*V2*T2
The resultant heat capacity will be the average of the capacities of the first and second fluids, weighted by volume(since it is a volumetric heat capacity rather than mass- or molar-specific):
- C(final) = (C1*V1 + C2*V2)/V(final)
And plugging this into the previous equation, we get:
- (C1*V1 + C2*V2)*T(final) = C1*V1*T1 + C2*V2*T2
- or
- T(final) = (C1*V1*T1 + C2*V2*T2)/(C1*V1 + C2*V2)
And either use this formula directly:
(4180*40*15 + 1925*30*70)/(4180*40+1925*30)
((4,180*40)*15+(1,925*30)*70)/(4,180*40+1,925*30) =29.1198
Or write a function if we want to repeat the calculation:
finalTemp2:=(c1,v1,t1,c2,v2,t2)->(c1*v1*t1 + c2*v2*t2)/(c1*v1+c2*v2)
Which we can then use like this:
finalTemp2(4180,40,15,1925,30,70)
finalTemp2(4,180, 40, 15, 1,925, 30, 70) =29.1198
Screenshot of KAlgebra after running these computations: